Direct Product Of Vector Spaces. The notion of map is determined by the category and this definition extends to other categories such as topological spaces. Have two vector spaces over C and the tensor product V W is a new complex vector space. More precisely let k be a field and I be an infinite set. Direct sum decompositions I Definition.

Let V be a finite-dimensional vector space such that U_1 U_2 U_m are subspaces of V and V U_1 oplus U_2 oplus U_m. Let U W be subspaces of V. Then mathrmdim V mathrmdim. The direct product or simply the product of two non-empty sets X and Y is the set X times Y consisting of all ordered pairs of the form xy x in X y in Y. X in X y in Y. The expansion is xy x0201y.
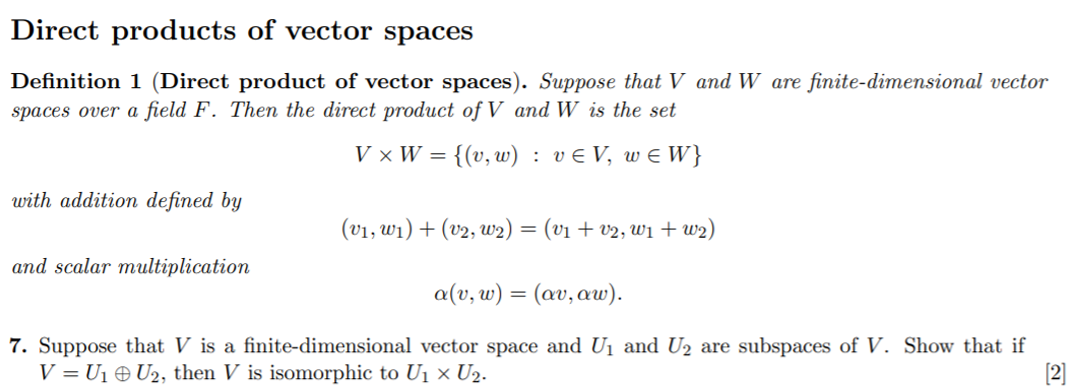
Then V U W if and only if for every v V there exist unique vectors u U and w W such that v u w.
Have two vector spaces over C and the tensor product V W is a new complex vector space. On the cartesian product V W fvw. This lecture provides concepts of direct product of two vector spaces and the criterion to be a subspace. The notion of map is determined by the category and this definition extends to other categories such as topological spaces. Let V be a finite-dimensional vector space such that U_1 U_2 U_m are subspaces of V and V U_1 oplus U_2 oplus U_m. For example the direct sum of n copies of the real line R is the familiar vector space Rn Mn i1 R R R 42 Orders of elements in direct products In Z 12 the element 10 has order 6 12 gcd1012.