
Determine The Kernel And Range. Let L be the linear transformation from M 2x2 to P 1 defined by. Then solving the system amounts to nding all of the vectors x 2Rn such that Tx 0. Range and kernel Let VW be vector spaces and L. Beginarrayltext a Lmathbfxleftx_3 x_2 x_.
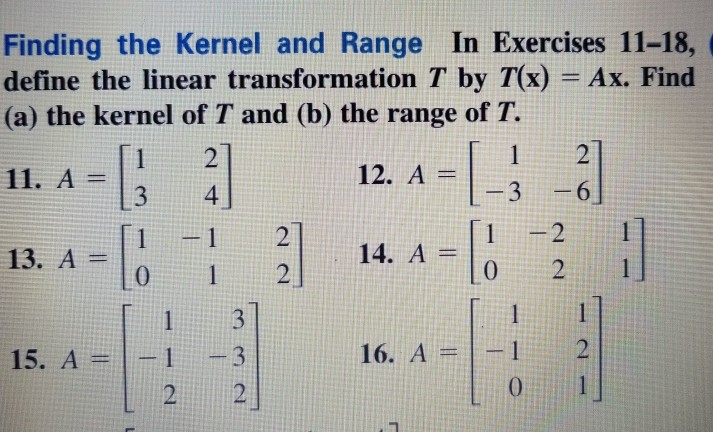
B Write down compatibility conditions on a b c for a solution to. Here we focus on finding the kernel range rank and nullity of a linear transformation. R3 R2 where Tx x1 x2 2x3T. V W be a linear transformation. To determine whether it is. T and rng T where T is the linear transformation given by.
The kernel is the same thing in the example youve given ie.
R2 R2 where Tx. By finding relations amongst the elements of LS Lv1 Lvn we can discard vectors until a basis is arrived at. The kernel is the same thing in the example youve given ie. View Answer Find bases for the kernel and range of the linear transformations T in the indicated exercises. A 1 1 3 5 6 4 7 4 2. The Kernel of denoted is the set of all points for which that is.